New nanologic machines consist of individual phosphorous atoms that are precisely positioned and embedded in a silicon crystal at a density of about 200 billion atoms per square centimeter. Single electrons randomly move in and out of the atoms due to quantum tunneling. Since each atom can hold one or two of these electrons, and each electron can occupy a few different energy levels, each atom can occupy one of four possible states. Each atom is constantly transitioning between its four states according to a certain set of probabilities, corresponding to the random movement of electrons tunneling in and out of the atom and changing their energy levels.
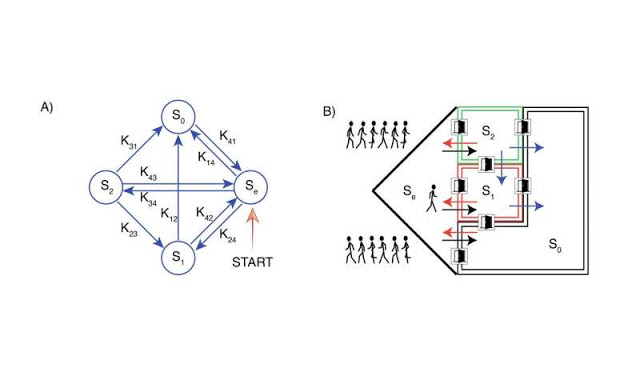
The researchers recognized that this physical picture can be used to simulate certain computational problems. As a proof-of-concept, they looked at a relatively simple example involving the flow of visitors in a maze consisting of four rooms connected by gates. The task is to find the optimal combination of rates for opening the gates in order to maximize the time that visitors spend in one particular room.
Solving this type of problem using conventional computing requires a significant amount of effort, since it typically involves analyzing the dynamics of visitors in the maze to gather information before attempting to optimize the rates of the gate openings.
However, using the new logic devices, it’s possible to find the solution more directly because the problem is physically embodied by the atomic “hardware” itself. For this particular problem, the topology of the maze corresponds to an atom’s states, and the movement of visitors corresponds to the tunneling of the electrons.
The researchers expect that the results will lead to nanoscale logic devices capable of solving a wide variety of problems of increasing complexity—all by directly simulating the problems rather than reframing them as binary processes.
“Nanoscale and molecular devices used as hardware for logic have potentially many advantages, from high packing density and low-power dissipation to the high number of states that can be used to encode information,” Remacle said. “However, their dynamics is ruled by probabilistic law because of the fundamental stochastic nature of thermally activated and quantum processes. The most straightforward application is then to use nanoscale devices for the implementation of probabilistic algorithms that require significant overhead in conventional deterministic hardware. For example, the mere sampling a pseudo-random number from a probability distribution requires hundreds of instructions on a modern computer while electron tunneling at truly random times is a natural process.”
In the future, the researchers plan to design other types of nanologic devices, whose implementation will require positioning the components with extreme precision.
Exploiting the potential of nanoscale devices for logic processing requires the implementation of computing functionalities departing from the conventional switching paradigm. They report on the design and the experimental realization of a probabilistic finite state machine in a single phosphorus donor atom placed in a silicon matrix electrically addressed and probed by scanning tunneling spectroscopy (STS). The single atom logic unit simulates the flow of visitors in a maze whose topology is determined by the dynamics of the electronic transport through the states of the dopant. By considering the simplest case of a unique charge state for which three electronic states can be resolved, they demonstrate an efficient solution of the following problem: in a maze of four connected rooms, what is the optimal combination of door opening rates in order to maximize the time that visitors spend in one specific chamber? The implementation takes advantage of the stochastic nature of electron tunneling, while the output remains the macroscopic current whose reading can be realized with standard techniques and does not require single electron sensitivity.
SOURCES- Nanoletters, PhysOrg

Brian Wang is a Futurist Thought Leader and a popular Science blogger with 1 million readers per month. His blog Nextbigfuture.com is ranked #1 Science News Blog. It covers many disruptive technology and trends including Space, Robotics, Artificial Intelligence, Medicine, Anti-aging Biotechnology, and Nanotechnology.
Known for identifying cutting edge technologies, he is currently a Co-Founder of a startup and fundraiser for high potential early-stage companies. He is the Head of Research for Allocations for deep technology investments and an Angel Investor at Space Angels.
A frequent speaker at corporations, he has been a TEDx speaker, a Singularity University speaker and guest at numerous interviews for radio and podcasts. He is open to public speaking and advising engagements.