Two groups report technical breakthroughs for trapped neutral atom quantum computers.
Optical engineering for sorting atoms into 3D patterns
Barredo and team report their use of precision optical-engineering methods to sort atoms into arbitrary 3D patterns.
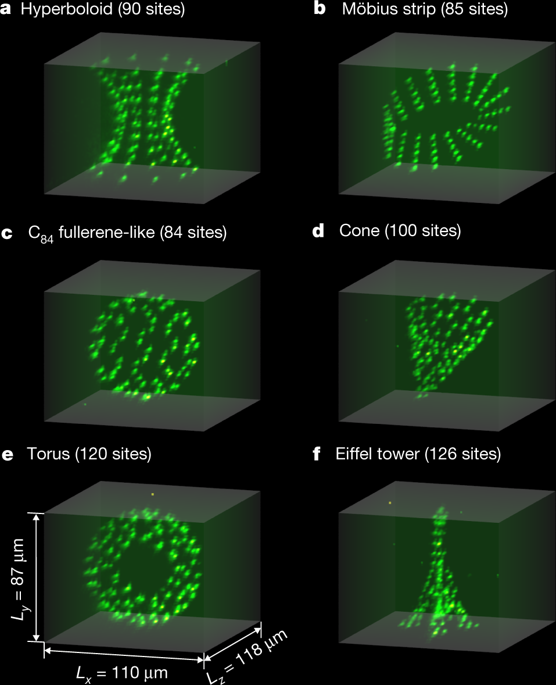
Barredo et al. extend their previously reported method for 2D atom sorting to three dimensions. Their approach to disorder and sorting is different from Kumar and colleagues’ method, but just as effective. They use a holographic technique whereby a laser beam is reflected off a spatial light modulator and then focused to form traps known as optical tweezers. In this way, they generate arrays of traps in arbitrary configurations that can be loaded with up to 72 cold rubidium atoms. To remove disorder and build the desired atomic configuration, the authors use a separate, movable optical tweezer to pluck atoms from ‘wrong’ traps and either move them to correct sites or discard them. This allows them to build qubit arrays in standard grid patterns, in topologies such as a Möbius strip, and even in the shape of the Eiffel Tower.

Cubic lattices and Maxwell’s demon
Kumar and his team constructed cubic lattices by revisiting a fanciful thought experiment known as Maxwell’s demon.

Above – A protocol for arranging neutral atoms in cubic optical lattices. Kumar et al. report a method for arranging ultracold, neutral caesium atoms in defined patterns in a cubic, 3D optical lattice — a series of laser-generated potential-energy wells in which atoms can be confined. Only one layer of atoms is shown, for simplicity. a, The atoms start off in random positions and in the same electronic state (state A, red). The shaded square indicates a target region that is to be filled with atoms. b, A combination of lasers and microwaves (wavy arrow) flips the state of one atom into a different state (state B, turquoise). c, A lattice shift is induced that moves the lattice and all atoms in state A half a step to the right and those in state B half a step to the left. d, The atom in state B is flipped back to state A. e, A reverse lattice shift moves the lattice and all atoms in state A half a step to the left, so that the square region is now filled with atoms.
The ability to organize neutral atoms exactly into planned 3D arrays will be valuable for the development of neutral-atom quantum computers that use a large number of quantum bits (qubits).
Arrays of isolated neutral atoms have long shown promise for quantum computing because neutral-atom qubits are extremely well isolated from environmental noise and are highly controllable, and also because such systems can be scaled up to large numbers of qubits. Given that controlled interactions between atoms are needed to perform quantum-computing operations, neutral-atom quantum computers will need qubits to be precisely arranged in a specified pattern. However, developing methods for sorting atoms into patterns has proved challenging. Neutral-atom qubits require ultracold temperatures and extremely high vacuums to function, and therefore require complicated apparatus; ordering them into arrays using optical techniques adds an extra level of practical complexity. Progress has been made in arranging neutral atoms in one and two dimensions, but 3D stacking will become essential as the number of qubits used approaches the hundreds, or to construct arrangements that have topologies not achievable in two dimensions.
Kumar’s team has extended their previously reported approach to assemble cold clouds of caesium atoms into a 3D lattice. The method begins with a randomly populated optical lattice: a trap formed from the interference patterns of counter-propagating lasers, in which atoms can be confined much like eggs in cartons. After imaging and recording the random locations of atoms in the lattice, the authors implement a sorting protocol that involves intricately controlling the polarizations of the lattice lasers, while using additional ‘addressing’ lasers and microwaves to position any given atom within a 5 × 5 × 5 array of lattice sites. In this way, up to 50 neutral atoms can be precisely ordered into an array that is suitable for use in a quantum computer.

Brian Wang is a Futurist Thought Leader and a popular Science blogger with 1 million readers per month. His blog Nextbigfuture.com is ranked #1 Science News Blog. It covers many disruptive technology and trends including Space, Robotics, Artificial Intelligence, Medicine, Anti-aging Biotechnology, and Nanotechnology.
Known for identifying cutting edge technologies, he is currently a Co-Founder of a startup and fundraiser for high potential early-stage companies. He is the Head of Research for Allocations for deep technology investments and an Angel Investor at Space Angels.
A frequent speaker at corporations, he has been a TEDx speaker, a Singularity University speaker and guest at numerous interviews for radio and podcasts. He is open to public speaking and advising engagements.