A new state of matter, called quantum spin liquid, produces long-range quantum entanglement which might help make quantum computers. They could enable topological qubits. Bill Gates and Microsoft have been funding efforts to create a topological quantum computer. Topological qubits could theoretically be far more stable and enable long lasting quantum calculations. The work is in early stages and is still basic research.
The theoretical advantage of a topological quantum computer based on trapped quantum particles is that the topological could be much more stable. Small, cumulative perturbations can cause quantum states to decohere and introduce errors in the computation, but such small perturbations do not change topological properties.
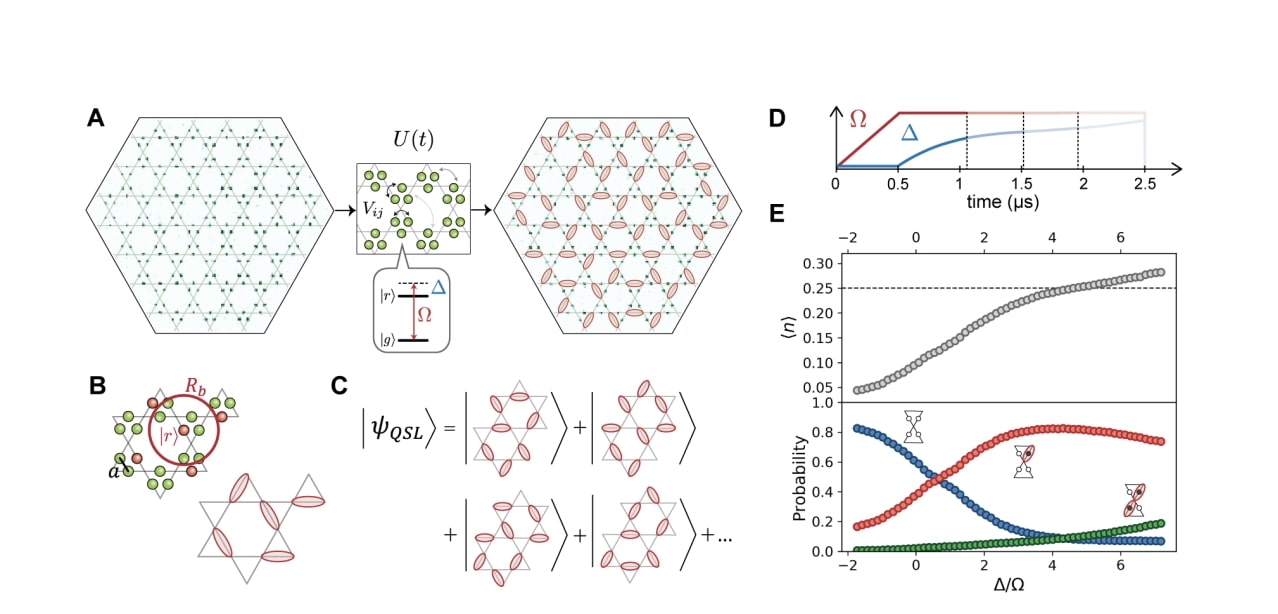
Above -Dimer model in Rydberg atoms arrays. A) Fluorescence image of 219 atoms arranged on the links of a kagome lattice. The atoms, initially in the ground state |gi, evolve according to the many-body dynamics U(t). The final state of the atoms is determined via fluorescence imaging of ground state atoms. Rydberg atoms are marked with red dimers on the bonds of the kagome lattice. (B) We adjust the blockade radius to Rb/a = 2.4, by choosing Ω = 2π × 1.4 MHz and a = 3.9 µm, such that all six nearest neighbors of an atom in |ri are within the blockade radius Rb. A state consistent with the Rydberg blockade at maximal filling can then be viewed as a dimer covering of the kagome lattice, where each vertex is touched by exactly one dimer. (C) The quantum spin liquid state corresponds to a coherent superposition of exponentially many dimer coverings. (D) Detuning ∆(t) and Rabi frequency Ω(t) used for quasi-adiabatic state preparation. (E) (Top) Average density of Rydberg excitations hni in the bulk of the system, excluding the outer three layers [28]. (Bottom) Probabilities of empty vertices in the bulk (monomers), vertices attached to a single dimer, or to double dimers (weakly violating blockade). After ∆/Ω ∼ 3, the system reaches ∼ 1/4 filling, where most vertices are attached to a single dimer, consistent with an approximate dimer phase.
Quantum spin liquid was first predicted by physicist Philip W. Anderson about 50 years ago, in 1973, but has never been observed in experiments until now.
The researchers used a “programmable quantum simulator,” a quantum computer that uses lasers to reproduce a physical setting and manipulate atoms in order to successfully recreate quantum spin liquid. The simulator allows them to position and shape atoms in any form they want.
A quantum spin liquid has magnetic properties, as its atoms become entangled and the material fluctuates and changes. While, in a normal magnet, all the electron spins align into large-scale patterns like the stripes of a checkerboard, quantum spin liquids have a third spin which creates a triangular pattern or lattice.
Quantum spin liquids could allow the creation of a “topological qubit” which stores information in the shape of a system, instead of in the state of a single particle.

Towards a topological qubit. To further explore the topological properties of the spin liquid state, they created an atom array with a small hole by removing three atoms on a central triangle, which creates an effective inner boundary. Various measurements and experiemnts represent the first steps towards initialization and measurement of a topological qubit.
These experiments offer unprecedented insights into elusive topological quantum matter, and open up a number of new directions in which these studies can be extended, including: improving the robustness of the QSL by using modified lattice geometries and boundaries as well as optimizing the state preparation to minimize quasiparticle excitations; understanding and mitigating environmental effects associated, e.g., with dephasing and spontaneous emission; optimizing string operator measurements using quasi-local transformations, potentially with the help of quantum algorithms. At the same time, hardware-efficient techniques for robust manipulation and braiding of topological qubits can be explored. Furthermore, methods for anyon trapping and
annealing can be investigated, with eventual applications towards fault-tolerant quantum information processing. With improved programmability and control, a broader class of topological quantum matter and lattice gauge theories can be efficiently implemented, opening the door to their detailed exploration.
This could be a new way to design of quantum materials that can supplement exactly solvable models and classical numerical methods.
Arxiv – Probing topological spin liquids on a programmable quantum simulator
Science – Probing topological spin liquids on a programmable quantum simulator
Synthesizing topological order
Topologically ordered matter exhibits long-range quantum entanglement. However, measuring this entanglement in real materials is extremely tricky. Now, two groups take a different approach and turn to synthetic systems to engineer the topological order of the so-called toric code type (see the Perspective by Bartlett). Satzinger et al. used a quantum processor to study the ground state and excitations of the toric code. Semeghini et al. detected signatures of a toric code–type quantum spin liquid in a two-dimensional array of Rydberg atoms held in optical tweezers. —JS
Abstract
Quantum spin liquids, exotic phases of matter with topological order, have been a major focus in physics for the past several decades. Such phases feature long-range quantum entanglement that can potentially be exploited to realize robust quantum computation. We used a 219-atom programmable quantum simulator to probe quantum spin liquid states. In our approach, arrays of atoms were placed on the links of a kagome lattice, and evolution under Rydberg blockade created frustrated quantum states with no local order. The onset of a quantum spin liquid phase of the paradigmatic toric code type was detected by using topological string operators that provide direct signatures of topological order and quantum correlations. Our observations enable the controlled experimental exploration of topological matter and protected quantum information processing.
Background on Microsoft Quantum and Topological Quantum Computers
Microsoft Quantum have been working on developing a topological qubit. The Microsoft qubit architecture is based on nanowires, which under certain conditions (low-temperature, magnetic field, material choice) can enter a topological state. Topological quantum hardware is intrinsically robust against local sources of noise, making it particularly appealing as we scale up the number of qubits.
An intriguing feature of topological nanowires is that they support Majorana zero modes (MZMs) that are neither fermions nor bosons. Instead, they obey different, more exotic quantum exchange rules. If kept apart and braided around each other, similar to strands of hair, MZMs remember when they encircle each other. Such braiding operations act as quantum gates on a state, allowing for a new kind of computation that relies on the topology of the braiding pattern.
Majorana zero modes (MZMs) localized at the ends of one-dimensional topological superconductors are promising candidates for fault-tolerant quantum computing. One approach among the proposals to realize MZMs—based on semiconducting nanowires with strong spin-orbit coupling subject to a Zeeman field and superconducting proximity effect—has received considerable attention, yielding increasingly compelling experimental results over the past few years. An alternative route to MZMs aims to create vortices in topological superconductors, for instance, by coupling a vortex in a conventional superconductor to a topological insulator.
Science – Flux-induced topological superconductivity in full-shell nanowires

Brian Wang is a Futurist Thought Leader and a popular Science blogger with 1 million readers per month. His blog Nextbigfuture.com is ranked #1 Science News Blog. It covers many disruptive technology and trends including Space, Robotics, Artificial Intelligence, Medicine, Anti-aging Biotechnology, and Nanotechnology.
Known for identifying cutting edge technologies, he is currently a Co-Founder of a startup and fundraiser for high potential early-stage companies. He is the Head of Research for Allocations for deep technology investments and an Angel Investor at Space Angels.
A frequent speaker at corporations, he has been a TEDx speaker, a Singularity University speaker and guest at numerous interviews for radio and podcasts. He is open to public speaking and advising engagements.